In this post we’ll discuss important theorem about parallelogram.
The theorem is very important and you have to remember it as it will help you solve variety of problems in your examination.
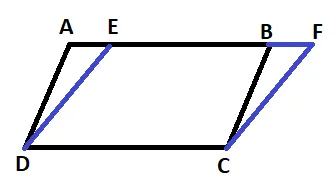
Consider the below parallelogram ABCD and EFCD.
Note that both the parallelograms have same base CD.
Also, both the parallelograms lie between same parallel lines AF & CD.

According to this theorem, if two parallelogram have same base and lie between the same parallel lines then they are equal in area.
i.e. Area ABCD = Area EFCD
I hope you got the basic context of the theorem, let us now try to prove it.
Proof of Parallelogram Theorem


Consider triangle DAE and triangle CBF;
( i ) ∠DAE = ∠CBF ( Corresponding angles )
Since DABC is a parallelogram, AD is parallel to BC and AB act like transversal.
So, ∠DAE = ∠CBF
(ii) ∠DEA = ∠ CFB
Since DEFC is a parallelogram, DE & CF are parallel lines & EF act as transversal.
So, ∠DEA = ∠ CFB
(iii) ∠ ADE = ∠ BCF
Since both the sides of triangles are equal to each other, then the third angle would also be equal.
We know that side opposite to equal angles are equal, so we can write;
AE = BF
Hence by ASA congruency condition, we can say that both triangles are congruent.
Triangle DAE ≅ Triangle CBF
Since both the triangles are congruent, it means that there areas are also equal.
So, area (DAE) = area (CBF)
Now let check if both the parallelogram ABCD and EFCD have same area.
The area of parallelogram ABCD can be written as;
Area (ABCD) = Area (DAE) + Area (EBCD)
we already proved that; Area (DAE) = Area (CBF)
So now the main equation can be written as;
Area (ABCD) = Area (CBF) + Area (EBCD)
Area (ABCD) = Area (EFCD)
Hence, both the parallelograms have same area.
So now we have proved the theorem that if two parallelogram have same base and lie between same parallel lines, it will both have same area.