Subscribe to our YouTube channel for the latest videos, updates, and tips.
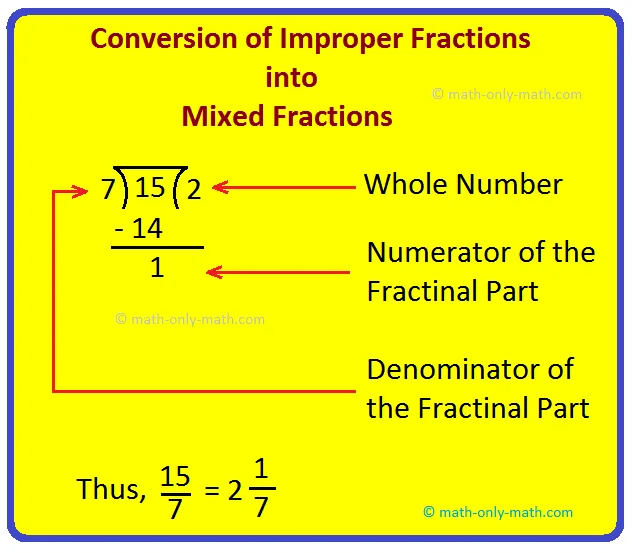
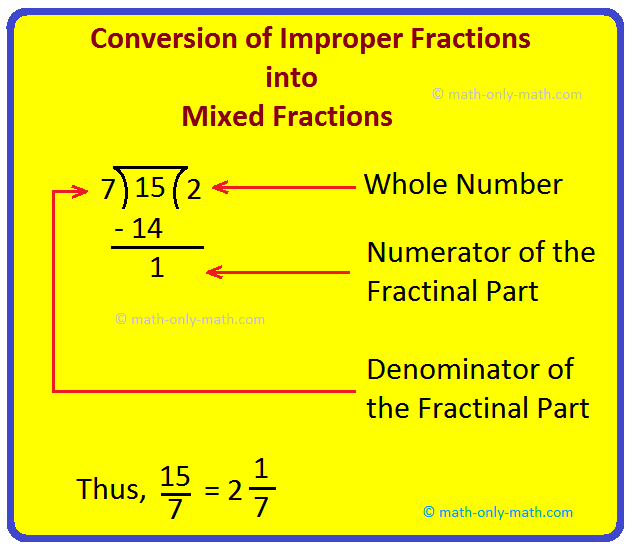
In conversion of improper fractions into mixed fractions, we follow the following steps:
Step I: Obtain the improper fraction.
Step II: Divide the numerator by the denominator and obtain the quotient and remainder.
Step III: Write the mixed fraction as: Quotient\(\frac{Remainder}{Denominator}\).
To convert an improper fraction into a mixed number, divide the numerator of the given improper fraction by its denominator. The quotient will represent the whole number and the remainder so obtained will be the numerator of the fractional part. The denominator of the fractional part will be the same as that of the improper fraction i.e.,
Conversion of Improper Fractions into Mixed Fractions Video:
Subscribe to our YouTube channel for the latest videos, updates, and tips.
Let us convert \(\frac{7}{5}\) into a mixed number.
As you know
if a fraction has same number as numerator and denominator, it makes a
whole. Here in \(\frac{7}{5}\) we can take out \(\frac{5}{5}\) to make a
whole and the remaining fraction we have is \(\frac{2}{5}\). So,
\(\frac{7}{5}\) can be written in mixed numbers as 1\(\frac{2}{5}\).
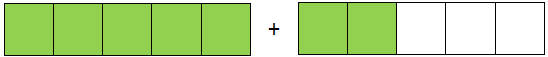
\(\frac{5}{5}\) = 1 + \(\frac{2}{5}\)
\(\frac{7}{5}\) = \(\frac{5}{5}\) + \(\frac{2}{5}\) = 1 + \(\frac{2}{5 }\) = 1\(\frac{2}{5}\)
|
Actually, \(\frac{7}{5}\) means 7 ÷ 5. When we divide 7 by 5 we get 1 as |
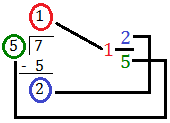 |
Examples on Conversion of Improper Fractions into Mixed Fractions:
For Example:
1. Express each of the following improper fractions as mixed fractions:
(i) \(\frac{17}{4}\)
We have,

Therefore, Quotient = 4, Remainder = 1, Denominator = 4.
Hence, \(\frac{17}{4}\) = 4\(\frac{1}{4}\)
(ii) \(\frac{13}{5}\)
We have,
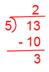
Therefore, Quotient = 2, Remainder = 3, Denominator = 5.
Hence, \(\frac{13}{5}\) = 2\(\frac{3}{5}\)
(iii) \(\frac{28}{5}\)
We have,
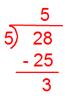
Therefore, Quotient = 5, Remainder = 3, Denominator = 5
Hence, \(\frac{28}{5}\) = 5\(\frac{3}{5}\).
(iv) \(\frac{28}{9}\)
We have,
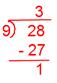
Therefore, Quotient = 3, Remainder = 1, Denominator = 9
Hence, \(\frac{28}{9}\) = 3\(\frac{1}{9}\).
(v) \(\frac{226}{15}\)
We have,
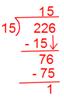
Therefore, Quotient = 15, Remainder = 1, Denominator = 15
Hence, \(\frac{226}{15}\) = 15\(\frac{1}{15}\).
2. Convert each of the following improper fractions into mixed numbers.
(i) \(\frac{15}{7}\)
(ii) \(\frac{24}{9}\)
Solution:
(i)
(ii)
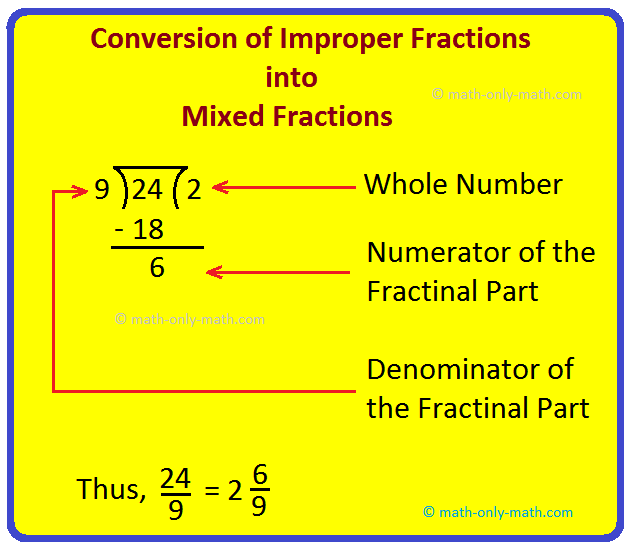
Conversion of an Improper Fraction Into a Mixed Fraction:
3. Let us convert \(\frac{22}{5}\) into an mixed fraction.
Divide the numerator 22 by the denominator 5.
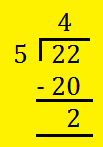
The quotient 4 gives the whole number. The remainder 2 is the numerator of the fractions.
The denominator of the fraction remains the same. So, \(\frac{22}{5}\) = 4\(\frac{2}{5}\)
4. Convert \(\frac{41}{3}\) into mixed fraction.
Divide the numerator 41 by the denominator 3.

The quotient 13 gives the whole number. The remainder 2 is the numerator of the fractions.
The denominator of the fraction remains the same.
So, \(\frac{41}{3}\) = 13\(\frac{2}{3}\)
Worksheet on Conversion of Improper Fractions into Mixed Fractions:
1. Convert the following into Improper Fractions:
(i) \(\frac{11}{9}\)
(ii) \(\frac{24}{5}\)
(iii) \(\frac{26}{8}\)
(iv) \(\frac{59}{9}\)
(v) \(\frac{64}{7}\)
Answer:
1. (i) 1\(\frac{2}{9}\)
(ii) 4\(\frac{4}{5}\)
(iii) 3\(\frac{2}{8}\)
(iv) 6\(\frac{5}{9}\)
(v) 9\(\frac{1}{7}\)
● Fraction
Representations of Fractions on a Number Line
Fraction as Division
Types of Fractions
Conversion of Mixed Fractions into Improper Fractions
Conversion of Improper Fractions into Mixed Fractions
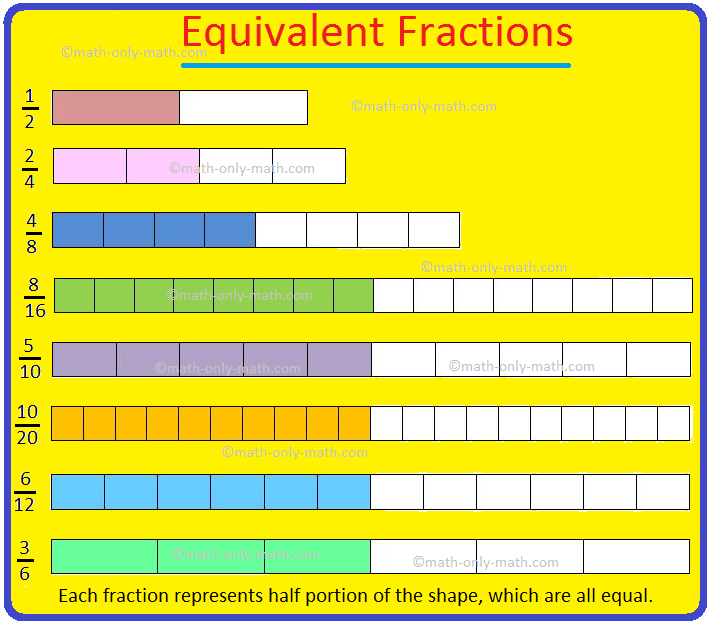
Equivalent Fractions
Interesting Fact about Equivalent Fractions
Fractions in Lowest Terms
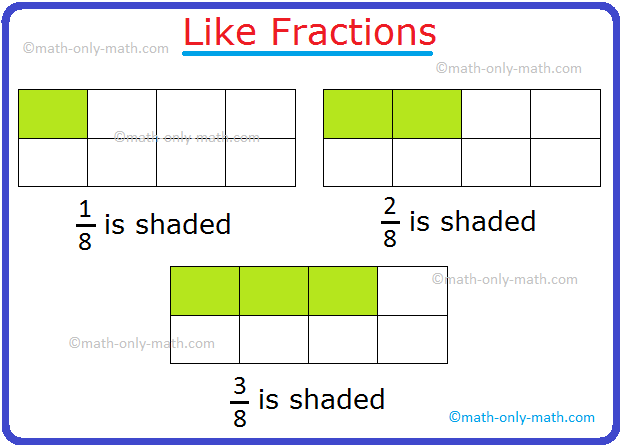
Like and Unlike Fractions
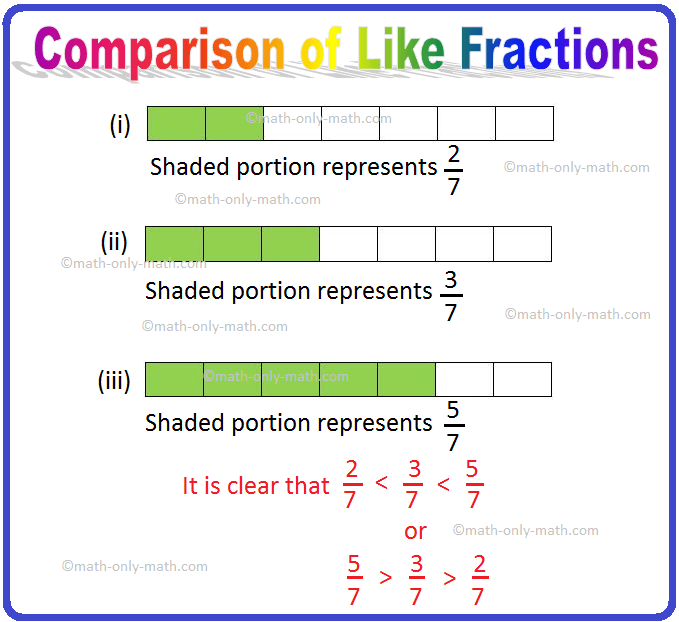
Comparing Like Fractions
Comparing Unlike Fractions
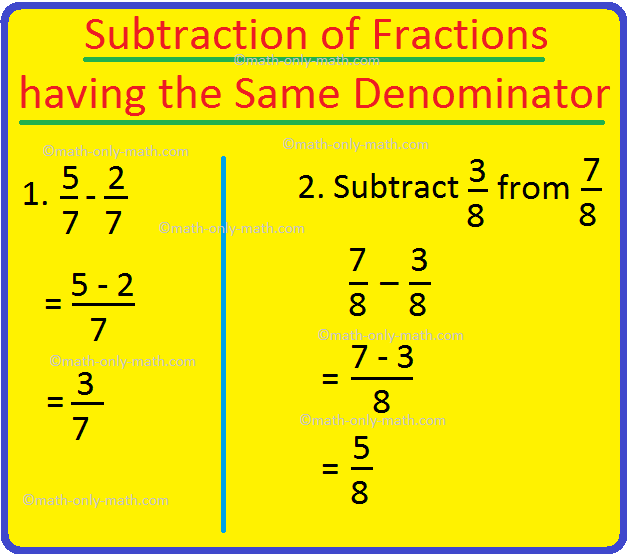
Addition and Subtraction of Like Fractions
Addition and Subtraction of Unlike Fractions
Inserting a Fraction between Two Given Fractions
Numbers Page
6th Grade Page
From Conversion of Improper Fractions into Mixed Fractions to HOME PAGE
Didn’t find what you were looking for? Or want to know more information
about Math Only Math.
Use this Google Search to find what you need.